[新しいコレクション] √3-2√2 is equal to 928674-What is 2 thirds equal to

For example, √2 √2 = 2 Here, √2 is an irrational number If it is multiplied twice, then the final

200以上 rationalize 2 √3/2-√3 129380-Rationalize the denominator 2+root 3/2-root 3

  I assume you want to rationalize the denominator You start with (1√2)/ (2√3) just multiply top and bottom by the conjugate of 2√3, which is 2√3 Now you have (1√2) (2√3) / (2√3) (2√3) = (1*2 2√2 1*√3 √6)/ (2^2 √3^2) = (2 2√2 √3 √6)/ (43) = 2 2√2 √3 √6 What you rationalize is the denominator of the fraction Eg 1/√2 is multiplied and divided by √2 to give √2/2 which is still irrational So you might be wondering what's the purpose of rationalising Let's do this, what is the value obtained if we add √2 √3 to 1/(√3 √2) It might be hard to tell right nowRD Sharma Solutions for Class 9 Maths Chapter 3 – Free PDF Download In Class 9, Rationalisation is one of the most important chapters RD Sharma Solutions for Class 9 Chapter 3 is about different algebraic identities and rationalisation of the denominatorA rationalisation is a process by which radicals in the denominator of a fraction are eliminated

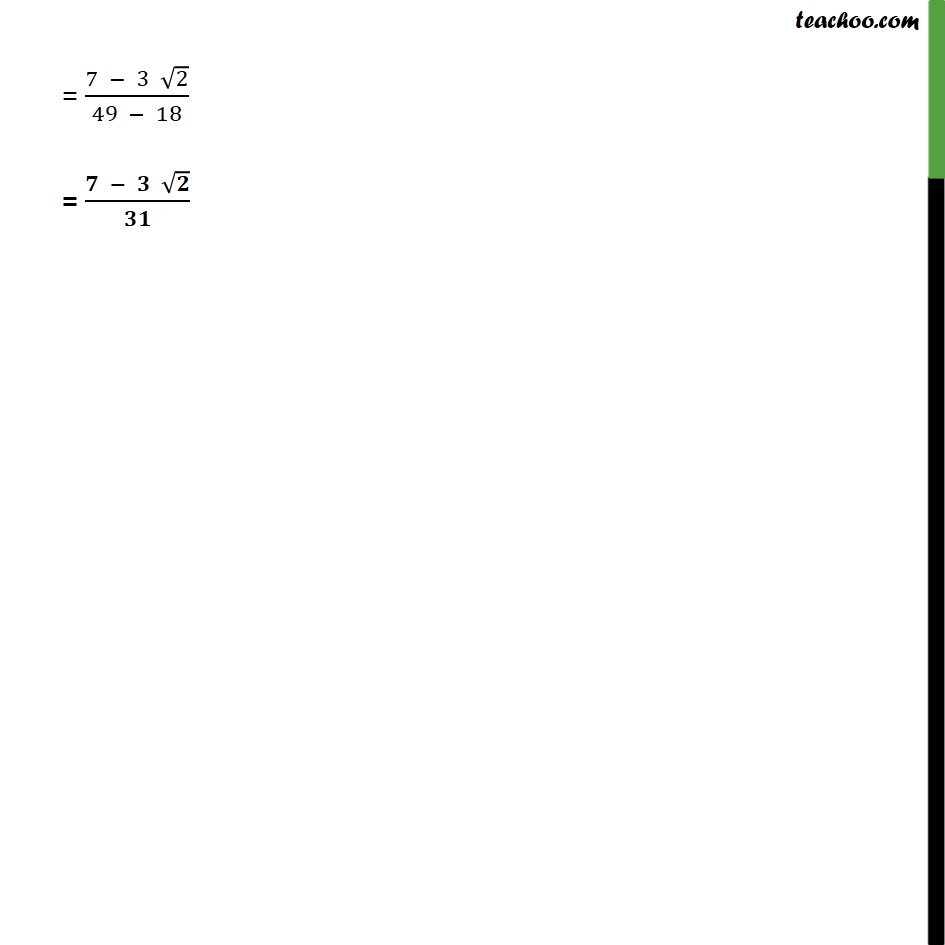
Example Rationalise The Denominator Of 1 7 3 Root 2

Rationalize the denominator 2+root 3/2-root 3